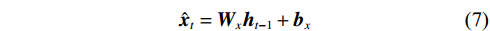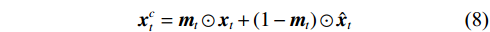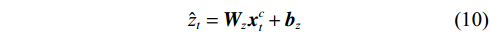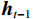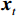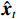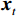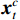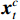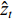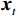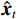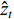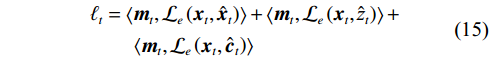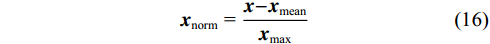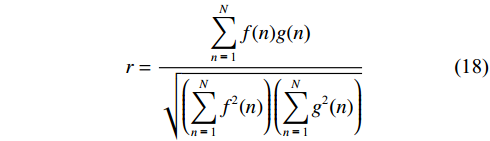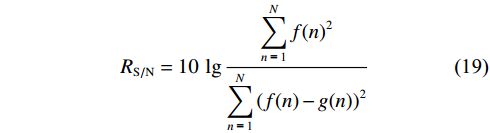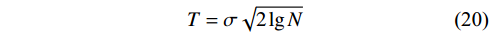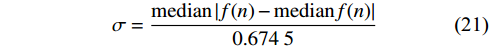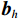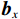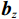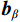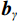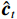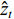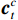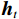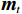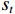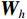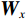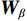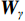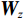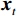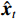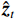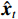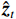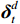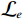Processing of impulse noise in magnetotelluric data based on bidirectional recurrent imputation
-
摘要:目的
大地电磁测深是一种通过观测天然电磁场获取地下电性结构的勘探方法,较易受到噪声干扰。脉冲类噪声是大地电磁工作中的常见噪声,其幅值高、频带宽,会对数据质量产生较大影响。
方法为了压制脉冲类噪声,以插补思想为基础,提出了基于时间序列双向循环插补模型(Bidirectional recurrent imputation for time series,BRITS)的大地电磁脉冲类噪声处理方法。首先,将噪声干扰段删除,此时大地电磁时间序列可视为待插补的缺失序列,而后利用该缺失序列构建训练集,对BRITS模型进行插补训练,训练完成后对缺失序列进行插补,即可得到去噪结果。通过仿真及实测含噪声数据处理,并与经验模态分解(Empirical mode decomposition,EMD)阈值方法进行了对比。
结果和结论结果表明:BRITS方法对仿真噪声数据处理后与原始数据的归一化互相关系数可达0.999以上,信噪比可达29 dB以上,EMD阈值方法处理前后相关系数为0.778,信噪比为3.09 dB;在实测数据处理中,BRITS方法有效恢复了噪声干扰数据,相比EMD阈值方法,其阻抗奈奎斯特图更接近天然大地电磁信号特征。通过不同训练样本试验得出:对4分量大地电磁数据而言,数据中至少需包含两道正常分量,单个含噪分量中噪声占比不大于20%,且噪声连续干扰长度不超过10个采样点,此时,BRITS方法去噪后数据的相关系数在0.96以上,可以保证一定的去噪精度。
Abstract:ObjectiveMagnetotelluric sounding is an exploration method to obtain the underground electrical structure by observing the natural electromagnetic field, which is easily disturbed by noise. Impulse noise, frequently occurring in magnetotelluric sounding, generally exhibits high amplitude and wide frequency bands, producing significant impacts on the data quality.
MethodsTo suppress such noise, this study proposed a method based on a bidirectional recurrent imputation for time series (BRITS) model. First, the data segments with noise interference were deleted. Second, for the magnetotelluric time series with missing data to be imputed, training sets were constructed for imputation training of the BRITS model. Third, imputation was conducted to supplement the missing data, yielding the denoising results. Last, the proposed method was applied to process the simulated and measured data with noise, and the application results were compared with the results derived using the empirical mode decomposition (EMD) threshold method.
Results and ConclusionsThe results of this study are as follows: (1) Relative to the original data, the simulated data with noise, after being processed using the BRITS method, manifested normalized cross-coefficient reaching up to 0.999 and signal-to-noise ratios of over 29 dB. In contrast, the simulated noise data, after being treated using the EMD threshold method displayed cross-coefficient of 0.778 and signal-to-noise of 3.09 dB. (2) In the processing of the measured data, the BRITS method effectively restored the data with noise interference, with the obtained Nyquist diagram closer to the characteristics of natural magnetotelluric signals compared to the EMD threshold method. (3) As indicated by the test results of different training samples, in the case where four-component magnetotelluric data contain at least two normal components, with the proportion of noise in a single unnormal component not exceeding 20% and the continuous noise interference length of 10 sampling points or less, the data denoised using the BRITS method can yield cross-coefficient exceeding 0.96, thus ensuring certain denoising accuracy.
-
大地电磁测深是一种以天然电磁场为场源的地球物理勘探方法,其凭借探测深度大、施工灵活便捷等诸多优势在矿产勘查、工程勘查、能源勘查等领域有着较为广泛的应用。但是,天然场信号一般比较弱,较易受到噪声干扰,所以,提高数据信噪比是大地电磁工作的重要研究课题。其中,脉冲类噪声一般幅值大,频带宽,如果不加以压制,它将严重影响数据质量甚至造成数据报废。针对脉冲类噪声的压制,学者们提出了多种方法。主要有时频域分解及阈值去噪方法,如基于小波变换与迭代回归技术的脉冲噪声压制方法[1-2]、基于EMD的空间滤波及阈值去噪方法[3]、基于EMD阈值的脉冲类噪声处理方法[4]以及阈值去噪方法[5],数学形态滤波方法[6-8],机器学习类方法[9-12]。除此之外,利用数学形态滤波与字典学习的组合方法也可以压制脉冲干扰[13]。以上方法中,小波函数的选取、滤波阈值的选取、数学形态滤波中的结构元素、字典学习和MP(mathching pursuit)方法中冗余字典的构造以及AR(auto regressive)模型的参数确定都具有一定的主观性和经验性,处理效果因人而异,限制了方法的推广,因此,如何在去噪中减少主观影响,利用数据驱动实现去噪是需要解决的问题。
近年来,人工神经网络技术凭借着拟合能力强、以数据驱动、主观因素影响小等优点在地球物理领域得到了广泛的应用[14-16],其在大地电磁时间序列噪声处理领域的应用基本集中在工频、方波等规则、连续性噪声的识别和处理等方面[17-20],而对于脉冲这类非规则、突变噪声研究较少。为减少主观因数影响,笔者提出了一种数据驱动的大地电磁噪声处理方法,拟利用时间序列双向循环模型(Bidirectional recurrent imputation for time series,BRITS),通过插补技术,用模型预测的数据替换脉冲噪声,提高数据的去噪精度,以确保数据的准确性和可靠性,为后续的地质解释和地球物理勘探工作提供更加可靠的数据支撑。
1 方法原理
1.1 时间序列缺失模式
定义多维时间序列为X={
${\boldsymbol{x}}_1^{}$ ,${\boldsymbol{x}}_2^{}$ , ···,${\boldsymbol{x}}_t^{}$ , ···,${\boldsymbol{x}}_T^{}$ },其中,${\boldsymbol{x}}_t^{}$ ∈RD,第t个观测数据xt包含了D个特征,${\boldsymbol{x}}_t^{}$ ={${{x}}_t^1, x_t^2, \cdots ,x_t^D$ }。综上,多维时间序列X可表示为:$$ {\boldsymbol{X}}{\text{ = }}\left[ {\begin{array}{*{20}{c}} {x_1^1}& \cdots &{x_T^1} \\ \vdots & & \vdots \\ {x_1^D}& \cdots &{x_T^D} \end{array}} \right] $$ (1) 在大地电磁外业数据采集中,由于各种不可控因素,经常会出现数据受到干扰或缺失的现象,将这些受损数据段删除后,大地电磁多分量时间序列就可以看作有缺失值(None)的多维时间序列。
便于后续处理,引入缺失时间序列的2个表征向量,即掩码向量和时延向量。
掩码向量定义为:
$$ {{m}}_t^d=\left\{\begin{split} & 0\qquad x_t^d\text{为}\mathrm{None} \\ & 1\qquad 其他\end{split}\right. $$ (2) 定义时延向量为:
$$\delta _t^d = \left\{\begin{split} & {{{s}_t} - {{s}_{t - 1}} + \delta _{t - 1}^d{\text{ }}}\qquad {t > 1,{m}_{t - 1}^d = 0} \\ & {{{s}_t} - {{s}_{t - 1}}}\qquad\qquad\; {{\text{ }}t > 1,{m}_{t - 1}^d = 1} \\ & {0\qquad\qquad\qquad\;\;\; {\text{ }}t = 1} \end{split} \right. $$ (3) 下式为缺失时间序列示例,采用间隔为2,数据采集时间为[0,2,4,6,8,10],
$$ {\boldsymbol{X}}_{{\mathrm{miss}}}=\left[ {\begin{array}{*{20}{c}} {\begin{array}{*{20}{c}} {11} & {13} & {{\mathrm{None}}} \\ {12} & {17} & {{\mathrm{None}}} \\ 8 & {{\mathrm{None}}} & {33} \end{array}} & {\begin{array}{*{20}{c}} {{\mathrm{None}}} & {23} & {16} \\ {{\mathrm{None}}} & {{\mathrm{None}}} & {32} \\ {19} & {16} & {{\mathrm{None}}} \end{array}} \end{array}} \right] $$ (4) 结合式(2)可计算出掩码向量为:
$$\boldsymbol{m}=\left[\begin{array}{*{20}{c}}1 & 1 & 0 & 0 & 1 & 1 \\ 1 & 1 & 0 & 0 & 0 & 1 \\ 1 & 0 & 1 & 1 & 1 & 0\end{array}\right] $$ (5) 结合式(4)和式(5)可得时延向量:
$$ {\text{δ}} = \left[ {\begin{array}{*{20}{c}} {\begin{array}{*{20}{c}} 0&2&2&4&6&2\\ 0&2&2&4&6&8\\ 0&2&4&2&2&2 \end{array}} \end{array}} \right] $$ (6) 1.2 BRITS模型
2018年,Cao Wei等[21]以单项不相关循环插补(Unidirectional uncorrelated recurrent imputation,RITS-I)及单项相关循环插补(Unidirectional correlated recurrent imputation,RITS)模型为基础提出了BRITS模型。该模型由数据驱动,通过正反向学习时间序列上下文及不同维度数据之间的关系进行缺失值插补,相比传统KNN(K nearest neighbors)及循环神经网络等方法精度有了显著提高。BRITS模型在空气质量、医疗[21]、气象[22-23]、雷达[24]等数据缺失值插补方面取得了良好的应用效果。
RITS-I模型的估计向量
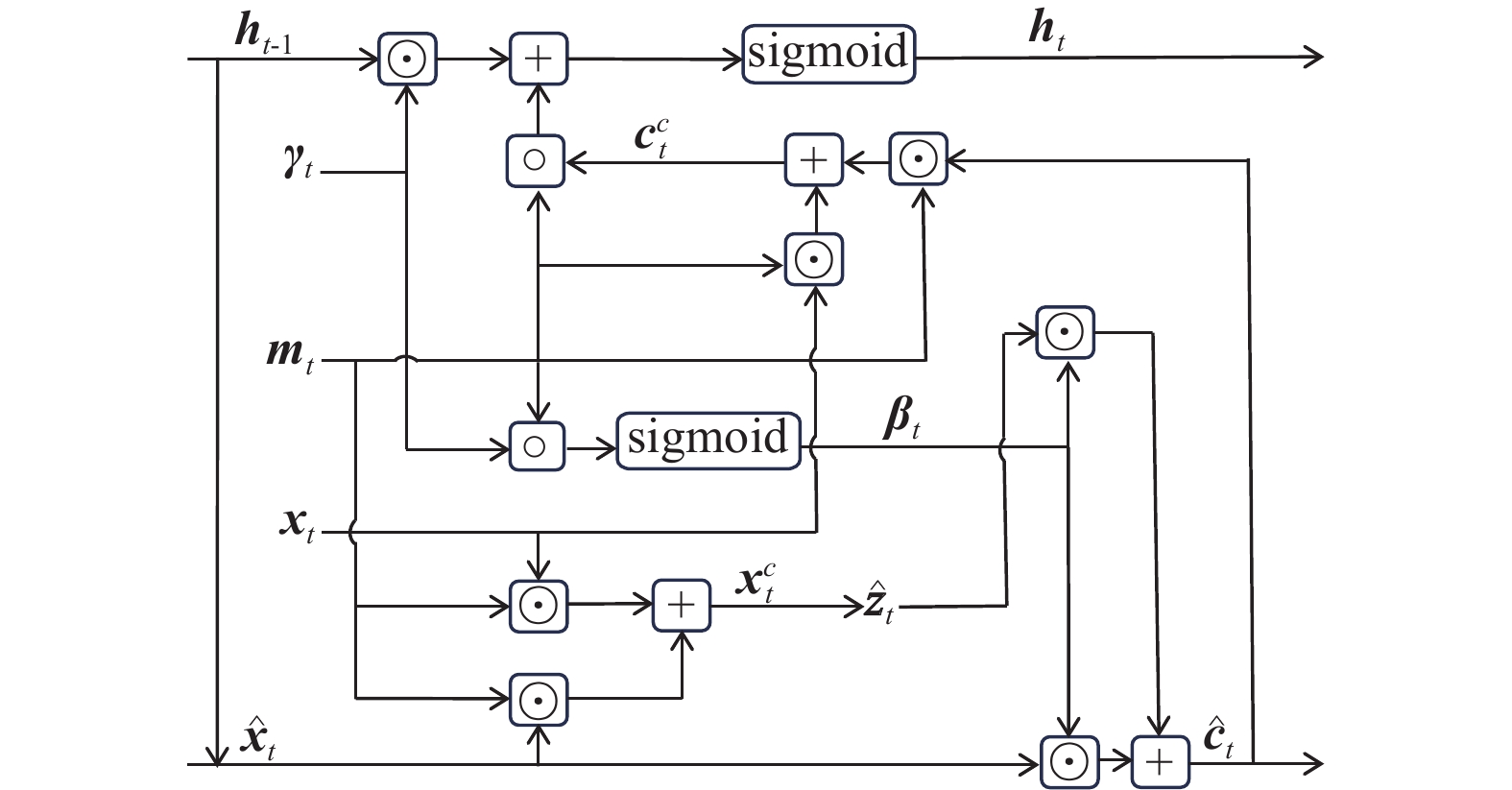
${\hat {\boldsymbol{x}}_t}$ 来自于历史观测值,与当前同时刻的不同时序特征无关。但大多数情况下,多维时序特征之间具有相关性,这种相关性也可以作为时间序列插补的重要依据,RITS模型在RITS-I的基础上考虑了特征之间的相关关系,进而提升了插补效果, RITS模型插补过程如图1所示。图2为RITS的运行逻辑结构图示,其具体工作流程如下:
$$ {\hat {\boldsymbol{x}}_t} = {{\boldsymbol{W}}_x}{{\boldsymbol{h}}_{t - 1}} + {{\boldsymbol{b}}_x} $$ (7) $$ {\boldsymbol{x}}_t^c = {{\boldsymbol{m}}_t} \odot {{\boldsymbol{x}}_t} + \left( {1 - {{\boldsymbol{m}}_t}} \right) \odot {\hat {\boldsymbol{x}}_t} $$ (8) $$ {{\text{γ}} _t} = \exp \left\{ { - \max \left( {0,{{\boldsymbol{W}}_\gamma }{{\text{δ}} _t} + {{\boldsymbol{b}}_\gamma }} \right)} \right\} $$ (9) $$ {\hat {{ {\textit{z}}}}_t} = {{\boldsymbol{W}}_{\textit{z}}}{\boldsymbol{x}}_t^c + {{\boldsymbol{b}}_{\textit{z}}} $$ (10) $$ {{\text{β}} _t} = \varphi \left( {{{\boldsymbol{W}}_\beta }\left[ {{{\text{γ}} _t} \circ {{\boldsymbol{m}}_t}} \right] + {{\boldsymbol{b}}_\beta }} \right) $$ (11) $$ {\hat {\boldsymbol{c}}_t} = {{{\text{β}}} _t} \odot {\hat {{\textit{z}}}_t} + \left( {1 - {{\text{β}} _t}} \right) \odot {\hat {\boldsymbol{x}}_t} $$ (12) $$ {\boldsymbol{c}}_t^c = {{\boldsymbol{m}}_t} \odot {{\boldsymbol{x}}_t} + \left( {1 - {{\boldsymbol{m}}_t}} \right) \odot {\hat {\boldsymbol{c}}_t} $$ (13) $$ {{\boldsymbol{h}}_t} = \varphi \left( {{{\boldsymbol{W}}_h}\left[ {{{\boldsymbol{h}}_{t - 1}} \odot {{{\text{γ}}} _t}} \right] + {{\boldsymbol{U}}_h}\left[ {{\boldsymbol{c}}_t^c\circ {{\boldsymbol{m}}_t}} \right] + {{\boldsymbol{b}}_h}} \right) $$ (14) 首先根据上一时刻即t−1时刻的隐藏层
${{\boldsymbol{h}}_{t - 1}}$ 得到t时刻时间序列${\boldsymbol{x}}_t^{}$ 的估计值,而后根据${\hat {\boldsymbol{x}}_t}$ 、${\boldsymbol{x}}_t^{}$ 和掩码向量${\boldsymbol{m}}_t^{}$ 获得补全向量${\boldsymbol{x}}_t^c$ ,由${\boldsymbol{x}}_t^c$ 又可得到t时刻包含特征属性的估计向量${\hat {{{{\textit{z}}}}}_t}$ ,其${{\boldsymbol{W}}_{\textit{z}}}$ 为对角元素为0的权重矩阵,这样可以建立每个特征与其他同时刻特征之间的关系。RITS模型的损失值由三部分组成,分别为实际观测时间序列值
${\boldsymbol{x}}_t^{}$ 与估计值${\hat {\boldsymbol{x}}_t}$ 、基于特征的估计向量${\hat {{{{\textit{z}}}}}_t}$ 和最终估计向量${\hat {\boldsymbol{c}}_t}$ 的平均绝对误差(Mean absolute error,MAE),这样可以同时兼顾时间域和同时刻不同特征的关系,进而提升训练效果和插补精度。$$ \begin{aligned} & {\ell _t} = \left\langle {{{\boldsymbol{m}}_t},{\mathcal{L}_e}\left( {{{\boldsymbol{x}}_t},{{\hat {\boldsymbol{x}}}_t}} \right)} \right\rangle + \left\langle {{{\boldsymbol{m}}_t},{\mathcal{L}_e}\left( {{{\boldsymbol{x}}_t},{{\hat {{\textit{z}}}}_t}} \right)} \right\rangle + \\ &\qquad \left\langle {{{\boldsymbol{m}}_t},{\mathcal{L}_e}\left( {{{\boldsymbol{x}}_t},{{\hat {\boldsymbol{c}}}_t}} \right)} \right\rangle \end{aligned} $$ (15) BRITS模型即为双向循环时间序列插补模型,以RITS模型为基础,分别对序列采取正向和反向的处理模式,同时兼顾时间序列多维分量之间的关系,可以得到更高的插补精度,本文所使用的即为BRITS模型。
1.3 处理流程
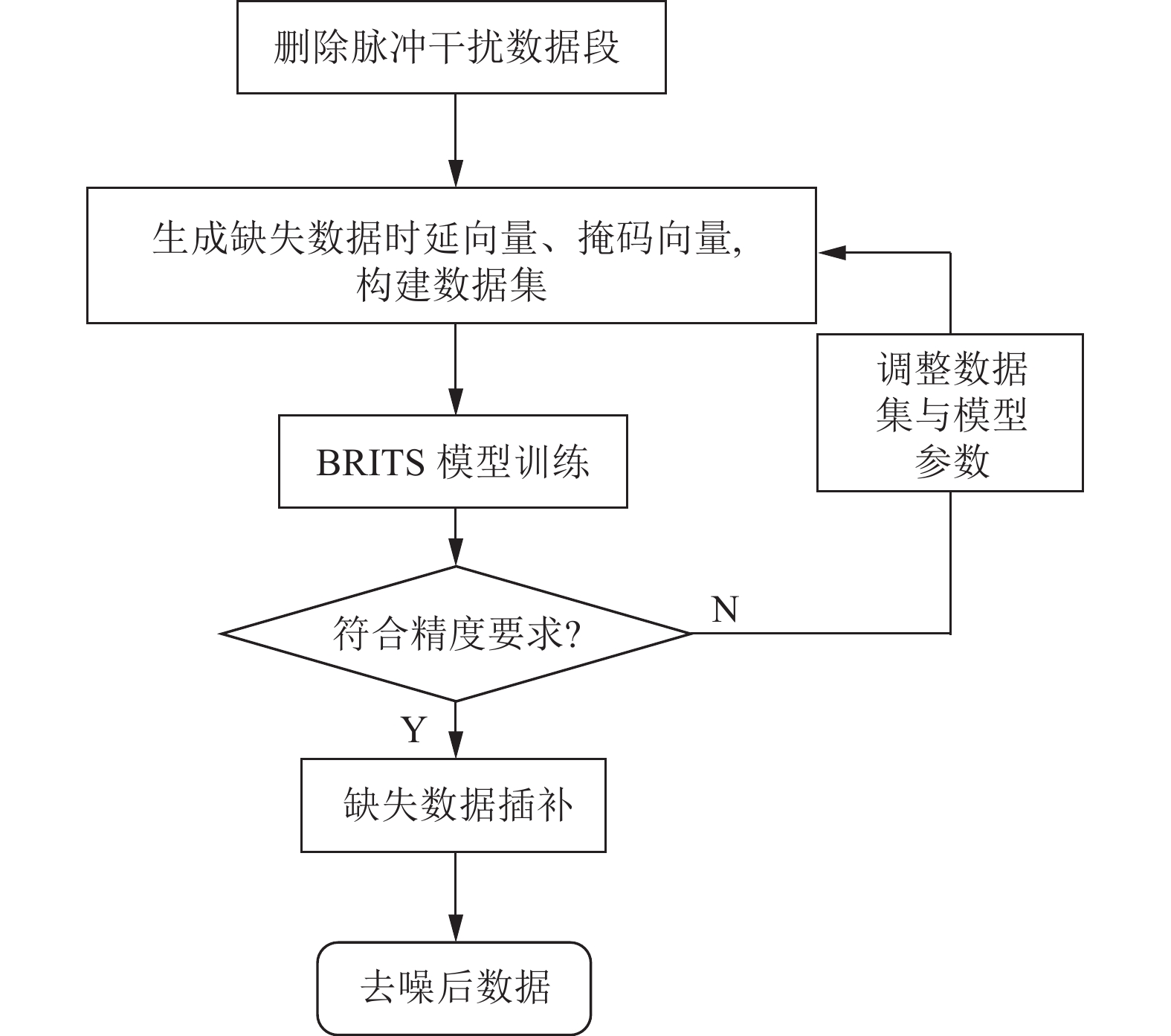
脉冲类噪声一般呈现出强度高、时间短、频带宽等特征,是比较常见的大地电磁噪声类型,一般出现在时间序列局部,通常在电场信号中比较常见。本文针对脉冲类噪声特征提出了基于BRITS的脉冲类噪声处理方法:首先,删除脉冲干扰数据段,利用BRITS模型学习大地电磁时间序列不同时刻和不同分量间的相互关系[25-26],而后进行删除段数据插补,从而实现脉冲类噪声的压制(图3)。
第一步:删除脉冲类噪声干扰时间序列数据段;第二步:计算缺失时序掩码向量与时延向量,构建数据集;第三步:训练BRITS时序插补模型;第四步:评价模型训练结果并调整数据集与模型参数,直至符合精度要求;第五步:使用BRITS插补缺失数据段;第六步:输出去噪后数据。
2 数据集构建与指标评价
2.1 数据集构建
首先删除时间序列脉冲类噪声干扰数据段,得到缺失序列,此处的大地电磁时序可以是一维的,也可以根据实际情况组合不同的电道或者磁道分量。训练数据集应包含缺失时间序列、掩码向量m以及时延向量δ,可以采取随机截取若干个长度的数据段作为一个训练样本来增大样本量,截取时序数据时同步截取掩码向量,再用截取到的掩码向量计算时延向量。
数据集时间序列数据均经过归一化(或标准化)处理,处理后的数据可以提高网络的收敛速度和网络精度,根据大地电磁时间序列数据的特征将其归一化:
$$ {{\boldsymbol{x}}_{{\text{norm}}}}{\text{ = }}\frac{{{\boldsymbol{x}}{{ - }}{{\boldsymbol{x}}_{{\text{mean}}}}}}{{{{\boldsymbol{x}}_{{\text{max}}}}}} $$ (16) 网络输出数据经反归一化,即可得到真实数据:
$$ {{\boldsymbol{y}}_{{\text{pred}}}}{\text{ = }}{{\boldsymbol{x}}_{{\text{max}}}}{{\boldsymbol{y}}_{{\text{norm\_pred}}}} + {{\boldsymbol{x}}_{{\text{mean}}}} $$ (17) 2.2 指标评价
本文选取网络理想输出时间序列和实际输出时间序列的归一化互相关系数(r)和信噪比(RS/N)作为检验网络精度的参数,具体计算如下:
$$ r=\frac{{\displaystyle\sum\limits_{n{\text{ = 1}}}^{{N}} {{f(}}n{{)g(}}n{\text{)}}}}{{\sqrt {\left(\displaystyle\sum\limits_{n{\text{ = 1}}}^{{N}} {{{f}}^{\text{2}}}(n)\right)\left(\displaystyle\sum\limits_{n{\text{ = 1}}}^{{N}} {{{g}}^{\text{2}}}{\text{(}}n)\right)} }} $$ (18) $$ {{R_{{\mathrm{S/N}}} = 10}}\;\lg \frac{{\displaystyle\sum\limits_{n{\text{ = 1}}}^{{N}} {{f(}}n{{)}}_{}^2}}{{\displaystyle\sum\limits_{n{\text{ = 1}}}^{{N}} {{\left( {{{f(}}n{{) - g(}}n{{)}}} \right)}^2}}} $$ (19) r值在−1到1之间,−1代表两组序列相位相反,0代表两组序列正交,1代表两组序列完全相同,r越接近于1,说明两组序列相似性越高(一般大于0.9)。
RS/N指信号和噪声功率之比,该参数越大说明两组信号之间越接近,一般大于10即可认为两组信号基本一致[27]。
3 参数选取
本文所有试验均在Pytorch 1.12.1上完成,CPU为Intel(R) Core(TM) i5-12500H 2.50 GHz,GPU为NVIDIA GeForce RTX 3050。
BRITS模型主要由输入层、循环层、回归层以及输出层构成(图1),其中循环层为该模型的主体结构,由若干长短时记忆网络神经元(Long short term memory cell,LSTMCell)构成,其主要参数为输入数据特征维度(input_size)和隐藏层维度(hidden_size),其中input_size要根据输入的大地电磁时间序列维度来确定,因本文所处理的大地电磁数据均由Ex、Ey、Hx和Hy共4个分量所构成,故模型的input_size设置为4,且文中所有试验的样本集均采用4分量。
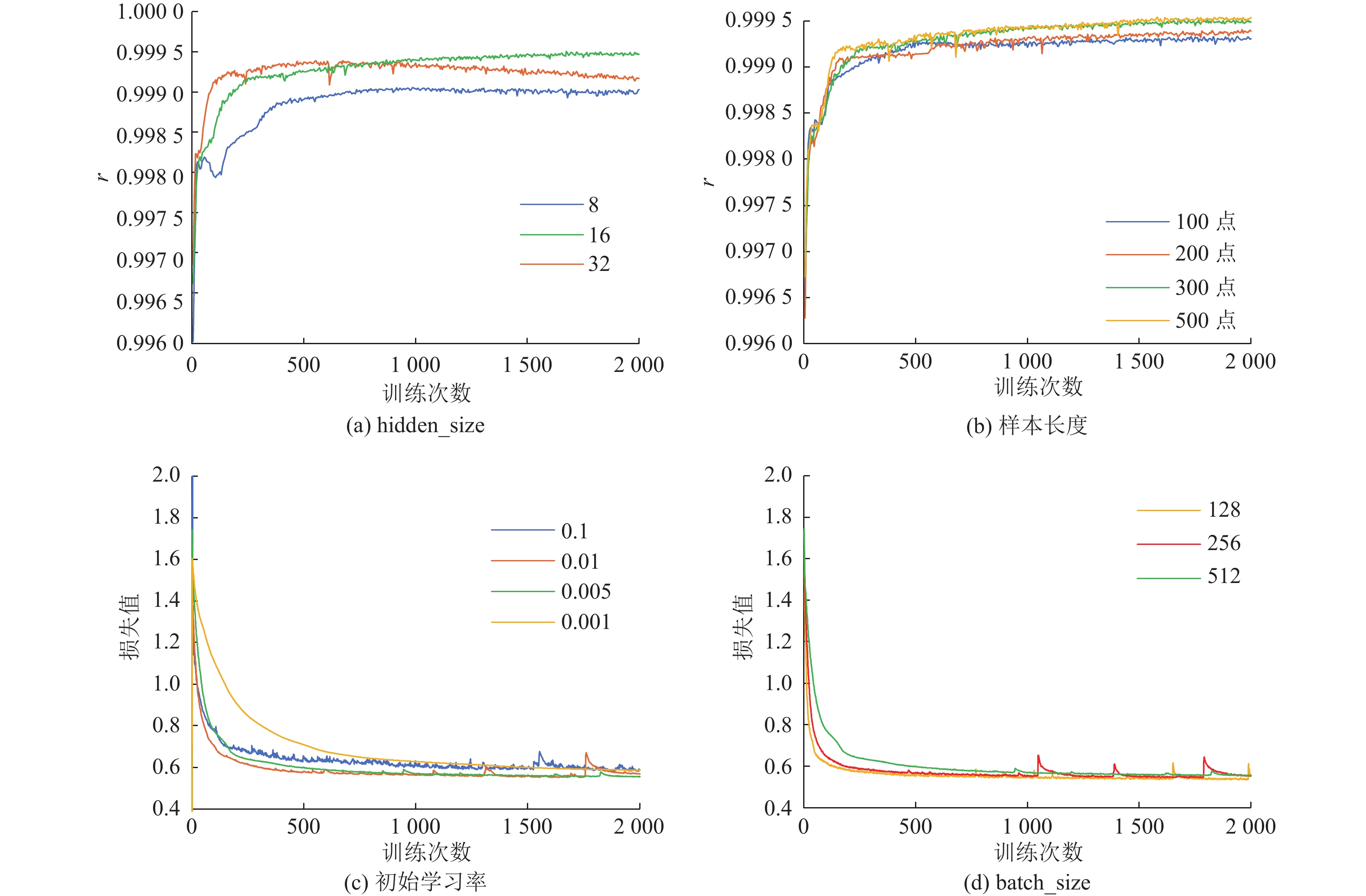
hidden_size需要根据数据的特征及复杂程度灵活选取,太大容易过拟合,太小对数据的特征表征不够全面,准确率降低。为选取适合大地电磁时间序列处理的隐藏层维度,分别对hidden_size取32、16、8的模型进行试验(图4a)。结果表明:当hidden_size为32时,模型在训练
1000 次后,r随着训练不升反降,出现过拟合;hidden_size为16时,r随着训练的进行逐渐增加,模型稳定收敛,不仅精度可以保证而且无过拟合现象;hidden_size为8时,虽然精度随着训练逐渐增加,但因特征维度过少,整体精度与hidden_size为16时差距明显。综上,本文BRITS模型的LSTMCell隐藏层特征向量维度设置为16。为了选取更合适的训练样本长度,分别对长度为100、200、300及500个点的样本数据进行试验。结果表明,样本长度一般与精度成正比,但样本过长将会增大内存开销与训练耗时。从图4b可知,当样本长度从100、200至300个点时,r随样本长度明显增大。当样本长度为500时,其精度增加与300时相比并不显著,但其内存开销增加了5%左右,训练时间则增加了近70%。所以,经过综合考量,本文样本长度均取300个点。
初始学习率是模型训练的重要参数,过大会导致收敛方向振荡,不易收敛,过小会造成模型收敛缓慢。本文分别对初始学习率取0.001、0.005、0.01与0.1时模型的损失值随训练的变化情况进行了试验(图4c)。由结果可知,当学习率为0.005时,模型既能保持稳定的收敛方向,又能达到一定的收敛速度,故将其作为本文模型训练的初始学习率。
batch_size表示模型训练中一次性输入的样本数量,过大会导致模型收敛缓慢,过小则会导致收敛方向振荡,训练耗时增加,在实际选取时可以在保证模型训练精度的情况下,尽可能利用内存,以稳定收敛方向加快训练速度。本文对batch_size取128、256和512进行试验,结果表明:小的batch_szie在一定程度上可以获得更小的损失值,但后期模型训练曲线波动较大,收敛方向不稳定,且训练更耗时(图4d)。考虑到本文数据量不大,本着提高模型训练速度与稳定模型收敛方向的原则,在充分利用硬件条件的基础上,本文将batch_szie设置为512。
为保证模型充分收敛,将训练轮次(epoch)设置为2 000,本文模型优化器使用适应性矩估计优化器(Adaptive moment estimation,Adam)。
4 实例分析
4.1 仿真数据
4.1.1 数据集构建
选取陕西旬阳某地采集的无明显人文干扰的大地电磁时间序列数据,该数据使用MTU-5A大地电磁仪采集,时长1 s,采样率2 400 Hz(图5a),在该数据段Ex及Ey通道上随机叠加仿真脉冲类噪声干扰,得到含有脉冲类噪声干扰的大地电磁时间序列(图5b),模拟脉冲噪声的振幅在原始信号中位数的15到25倍之间随机取值,干扰连续长度随机分布在5个采样点以内。文中幅值单位count表示仪器的模数转换(Analog-to-digital converter,ADC)值。
在删除干扰数据时,如果数据量不大,可以采用人工识别删除脉冲类噪声数据段,精准度高。如果数据量较大可以根据信号特征设置噪声截断阈值以批量化删除。本文主要以阈值截断的方式进行干扰段的识别和删除,采取的噪声阈值[4]表示为:
$$ T = \sigma \sqrt {2\lg N} $$ (20) $$ \sigma = \frac{{{\mathrm{median}} \left| {f(n) - {\mathrm{median}}f(n)} \right| }}{{0.674\;5}} $$ (21) 当大地电磁时序的绝对值|f(n)|大于阈值T时,将该时刻的数据删除并标记为缺失值(图5b),处理后Ex的缺失率为1.63%,Ey的缺失率为1.17%,删除干扰段后使用缺失的Ex、Ey以及原信号Hx及Hy道数据共同组合为一个长度2 400个点、共4个特征维度的多维时间序列数据。然后,随机截取长度300个点为一个训练样本,同时计算掩码向量m与时延向量δ,产生训练样本2 560个。需要指出的是,由于BRITS为数据驱动的插补模型,训练数据即为需要处理的插补数据,故该模型不设置验证集,训练好后直接进行数据插补。
4.1.2 模型训练
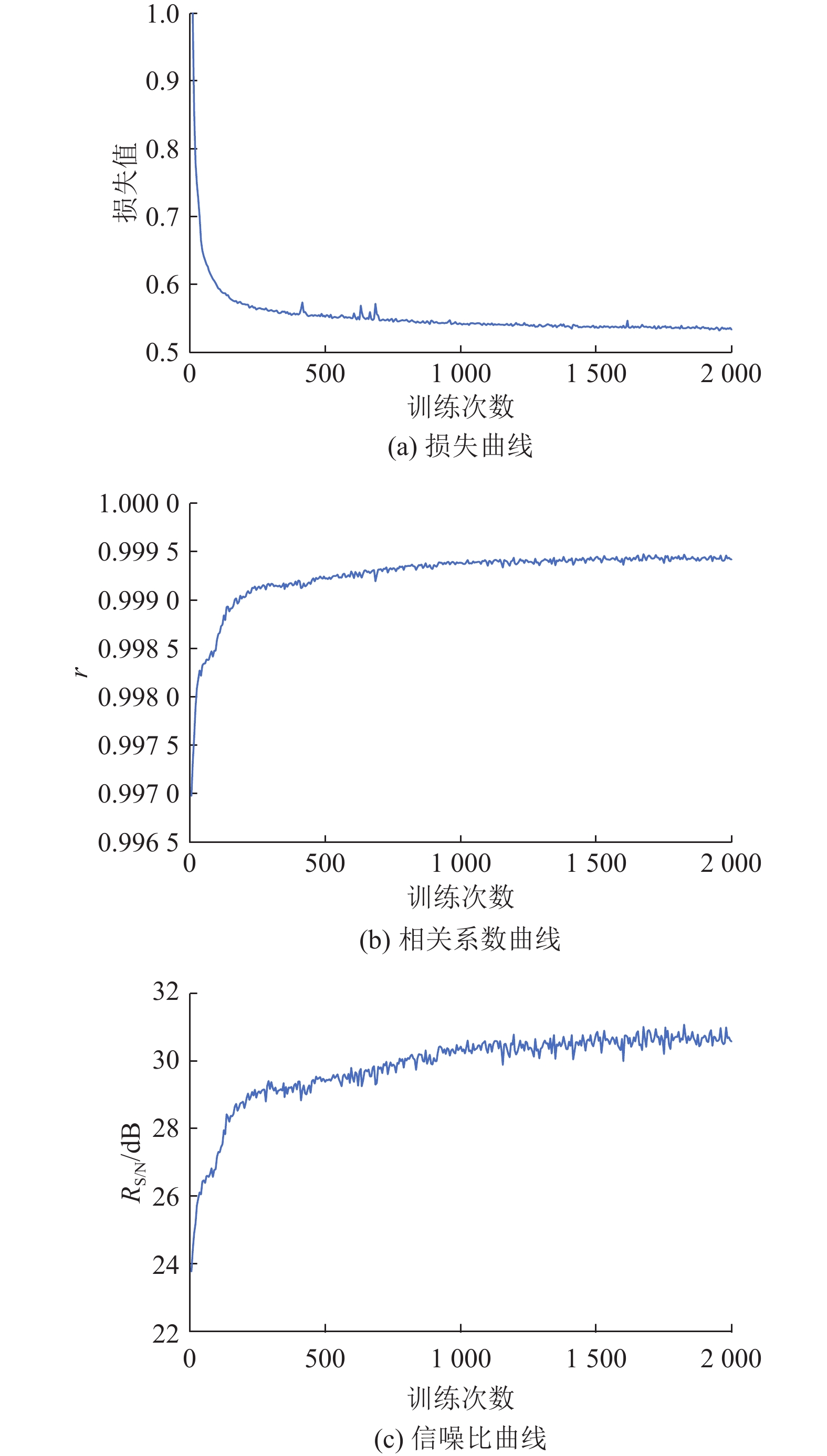
从训练曲线来看(图6):前500次训练模型损失快速下降,r及RS/N快速上升;500至
1500 次训练损失下降逐渐平缓,r及RS/N缓慢增加;1500 次训练后,网络逐渐接近收敛,损失不再明显减小,r及RS/N也不再明显增加,此时r可达0.9994 以上,RS/N可达30 dB以上,说明模型经过训练后可以准确地学习大地电磁不同分量及同一分量之间的关系,这样就完成了缺失数据的插补。根据训练效果选取1 800次训练模型进行下一步仿真数据处理。
4.1.3 处理效果分析
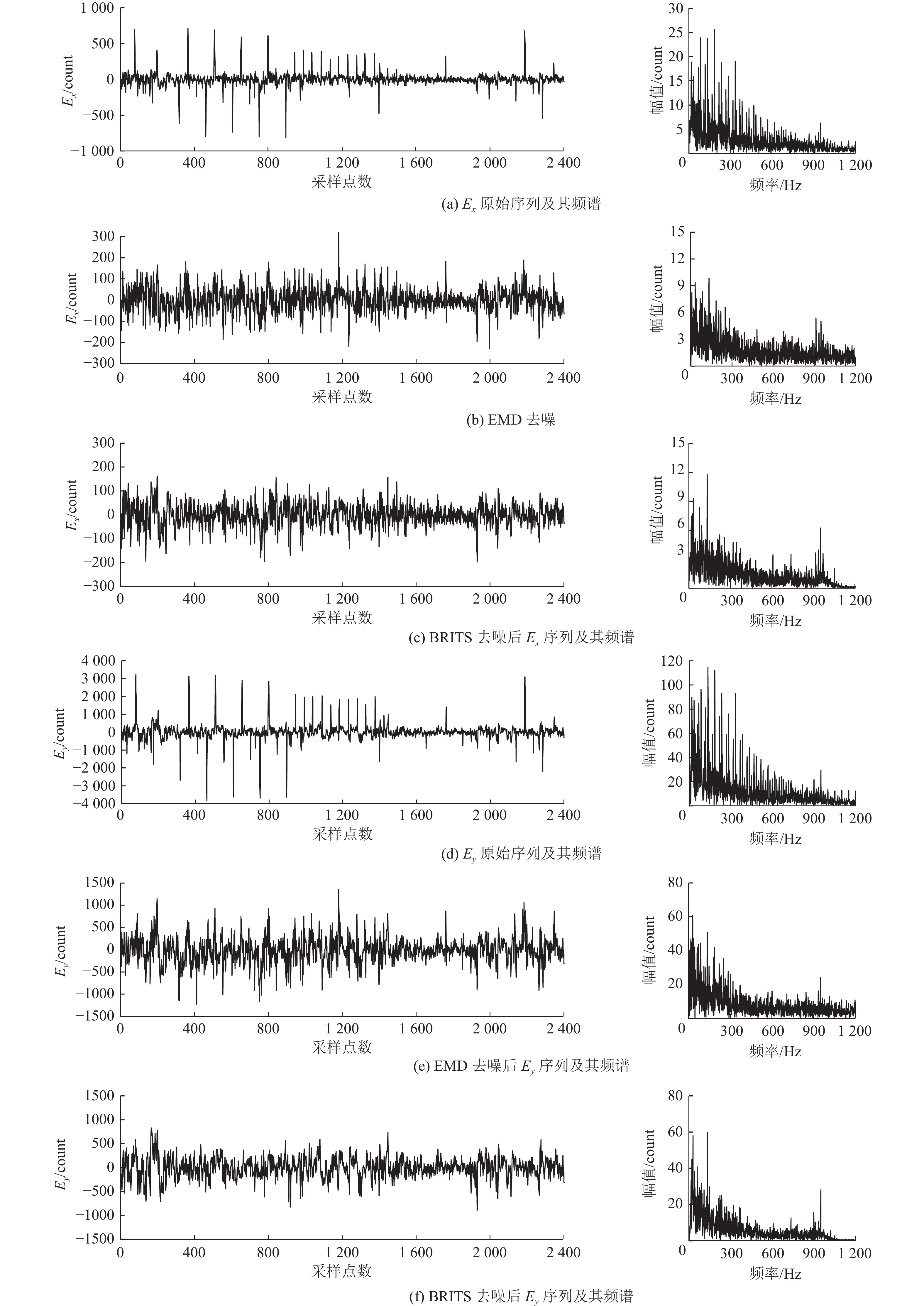
为了进一步检验本文方法的有效性,将BRITS方法与传统的EMD阈值去噪方法[4]处理结果(图7、图8)进行了对比。其中,添加仿真脉冲类噪声后,Ex及Ey时序波形被严重干扰,相比原始数据其频谱也明显增强,且影响频带较宽(图7b、图8b)。使用EMD及BRITS方法去噪后,尖峰脉冲类噪声已经基本消失,频谱中噪声的能量也明显减小,但是从时域波形细节来看,EMD方法还有些许噪声残留,且频谱相对于原始数据有明显的能量增加(图7c、图8c),相比EMD方法本文方法去噪则更为彻底,时域波形及频谱特征都更接近于原始数据(图7d、图8d)。
由表1可知,未处理前仿真数据r为0.602,RS/N为−2.10 dB,EMD阈值方法处理后一定程度上提升了数据质量,本文方法处理后数据精度提升则更为明显,初步展现了利用插补思想处理短时脉冲类噪声的优势。
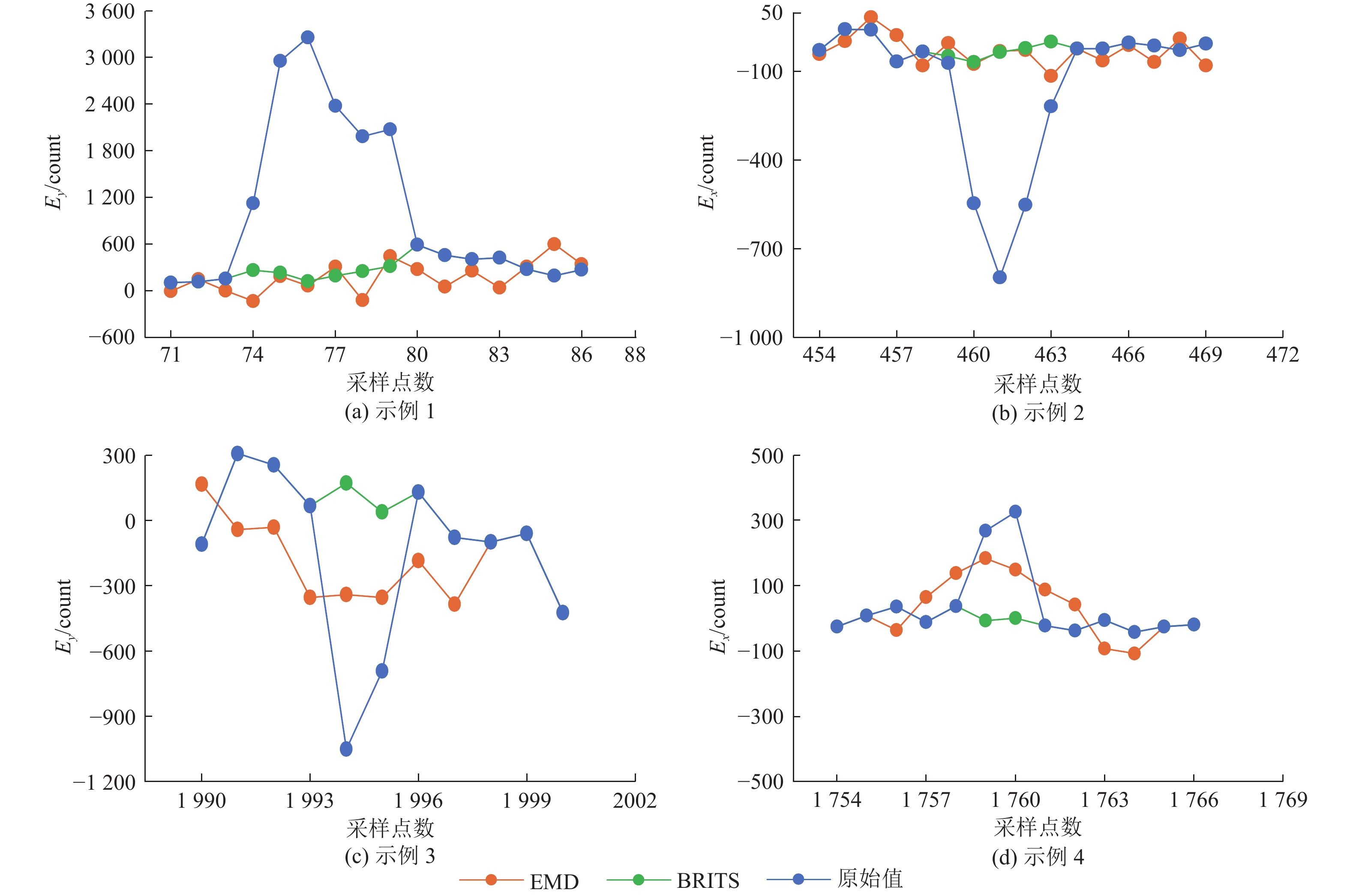
表 1 仿真数据精度对比Table 1. Comparison of accuracy of different denoising methods for simulated data类型 r RS/N/dB 仿真数据 0.602 −2.10 EMD阈值方法 0.778 3.09 BRITS方法 0.999 29.97 从时间序列(图9)来看,经BRITS模型处理后,干扰数据段得到了很好恢复,干扰点插补数值及总体波形与原始序列较为接近。而EMD阈值方法去噪后噪声附近的波形与原始波形有不小偏离,这是由于阈值去噪类方法在计算阈值时很难精确地寻找到信号和噪声的边界点,尽可能地在抑制噪声的同时保留有效信号,这就造成噪声附近的有效信号被“误伤”。而本文所应用的插补思想只对噪声干扰段进行处理,处理范围更小,针对性更强,可以在去噪的同时尽可能保留更多的原始信号。
从阻抗结果看,添加模拟噪声后阻抗变得极不稳定,多个频点阻抗数据发生严重偏离。其中,xy方向视电阻率及阻抗相位在9.4、11.2、49、97、265、320 Hz等多个频点出现较大偏移(图10a、图10b);yx方向视电阻率曲线在小于22.5 Hz的频点出现明显上移,在66、229、265 Hz 等频点处也有严重波动(图10c),yx阻抗相位在97和265 Hz频点处出现较大偏移,其余频段稳定性也欠佳(图10d)。经EMD阈值方法去噪后,阻抗稳定性只得到了有限的提升,且与原始阻抗一致性不佳,特别是xy方向视电阻率曲线在57至265 Hz频段之间明显下移,说明在去噪的同时损失了一部分该频段的有效信号;BRITS方法处理后阻抗曲线偏移频点均得到恢复,且与原始阻抗重复性良好,进一步说明了本文所提方法的正确性和可靠性。为了进一步检验该方法实际去噪能力,将使用实测含噪大地电磁数据进行处理试验。
4.2 实测数据
4.2.1 数据集构建
选取陕西旬阳某地采集的具有典型脉冲类噪声的大地电磁时间序列段,时长1 s,采样率2 400 Hz,该数据段Ex及Ey分量因附近用电设备的干扰,出现了一系列脉冲类噪声(图11a),如果不加以处理将严重影响数据后续处理工作。
首先使用阈值截断法得到缺失Ex、Ey分量时间序列(图11b),截断后Ex分量缺失率为3.96%,Ey分量缺失率为3.92%,与原Hx及Hy通道数据组合为一个长度2 400个点,4个特征维度的多维时间序列数据。以300个点为单位随机截取训练样本,同时计算掩码向量m与时延向量δ,共产生样本2 560个。此外,为了进行模型验证,选取该实测数据连续长度为311个采样点的无脉冲类噪声干扰段,构建缺失序列,缺失率分布在10%~15%之间,连续缺失长度控制在10个点以内。而后,随机截取长度为200个点的数据段作为一个验证样本,共生成验证样本1 280个。
4.2.2 模型训练及验证
从损失曲线特征看,前500次训练损失快速下降,之后下降趋势逐渐变缓,1 500次训练后损失逐渐稳定,模型趋于收敛(图12a)。图12b、图12c为验证集数据在各训练模型上的r和RS/N曲线,可知前500次随着训练的进行,模型快速提取了数据特征并完成插补,r及RS/N不断增高,r最高可达0.96以上,RS/N最高达10.45 dB以上,500次训练后r及RS/N不增反降,呈现出了过拟合的特征,故取训练500次的模型作为下一步数据插补的最终模型。
4.2.3 处理效果分析
原始数据Ex及Ey分量时序波形包含了多个幅值较高的尖峰状脉冲类干扰,其幅值可达有效信号的数十倍,频谱也表现出了脉冲类噪声的多级次谐波频谱特征,几乎覆盖了所有频段(图13a、图13d)。经EMD阈值及BRITS方法去噪后,Ex及Ey中的脉冲类噪声被基本压制,时序波形恢复了非平稳性和随机性[28-29],两者频谱中的脉冲各级次谐波也得到了显著抑制,有效信号频谱更加突出,但从时域波形及频谱细节看,EMD方法波形中仍然有一些细小的尖峰状脉冲噪声存在,且其频谱能量也明显高于BRITS方法的(图13b、图13c、图13e、图13f)。
从去噪前后局部时序波形看,2种方法均能有效削减噪声,但用EMD方法去噪时对相邻数据的影响是不可避免的,而BRITS方法能在去噪的同时能更完整地保留相邻数据,且插补的数据与相邻数据特征连续,波形相符,噪声抑制更精细(图14)。
从阻抗结果看,由于噪声的影响,原始阻抗极不稳定,在49 、66、132、159、229 Hz等众多频点处视电阻率和阻抗相位均出现了严重的偏离和波动,严重影响下一步处理工作。在EMD阈值去噪后,阻抗质量得到了一定程度的提高,但在xy方向的49、97、229 Hz等频点及yx方向18.8、49、115 Hz等频点仍有不小偏离。经过本文方法处理后,阻抗曲线则更为稳定和连续,各“飞点”得到了较好的抑制(图15),阻抗质量得到了明显提升。为了进一步评价去噪效果,引入阻抗的奈奎斯特图[30-31],在无噪声干扰条件下,阻抗的奈奎斯特图一般按频率呈顺时针或逆时针连续规则展布,如果受到噪声干扰,频点则会偏离这种分布趋势。由于噪声的影响,原始数据的奈奎斯特图呈杂乱无章的状态,其中,33、49、66、132、159 Hz等多个频点均有较大偏离(图16a、图16b);EMD阈值处理后,奈奎斯特图的分布范围有了明显减小,但其分布趋势仍然不太明显,依然比较杂乱(图16c、图16d);本文方法处理后,Zxy奈奎斯特图随频率增大呈现出了明显的顺时针分布趋势,Zyx则随频率呈逆时针分布趋势,比较接近天然大地电磁信号的特征(图16e、图16f),说明干扰得到了充分抑制,数据信噪比得到了显著提升。
综上,相比传统的EMD阈值方法,本文所提的基于BRITS模型的插补处理方法在去噪精准度、有效信号保留能力上更具优势,去噪具有更强的针对性。此外,利用BRITS模型可以提取大地电磁不同分量及不同时刻之间关系特征的优势,可以将其推广至其他与脉冲类噪声特征相似的短时间、突变性的噪声处理中。
5 讨 论
1) 脉冲类噪声占比影响
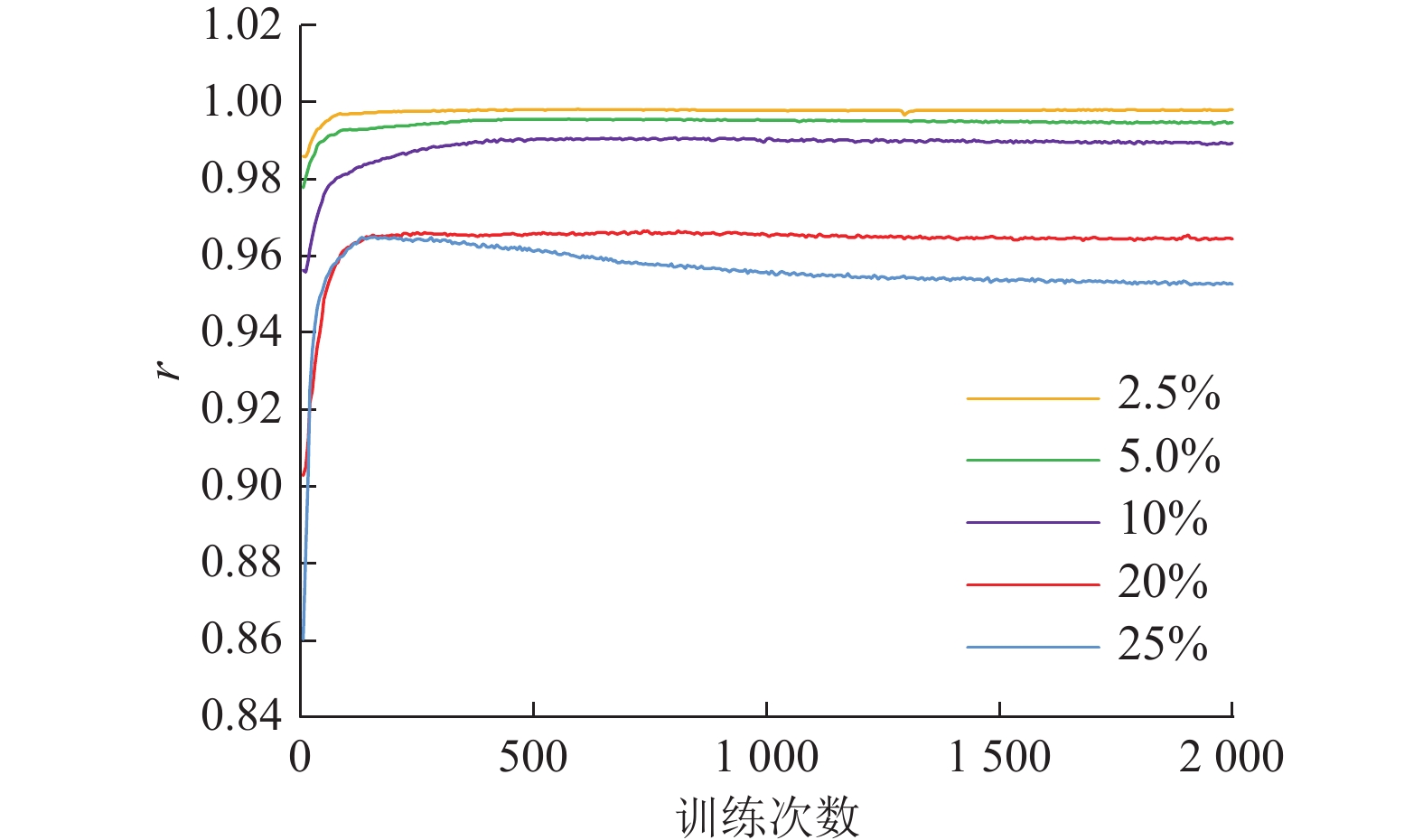
大地电磁信号中脉冲类噪声所占的比例(数据缺失率)是影响BRITS方法去噪效果的重要因素。为此,本文分别对缺失率为2.5%、5%、10%、20%与25%的样本进行了试验,各样本中连续干扰长度随机分布在10个采样点以内,且假设脉冲类噪声只存在于Ex及Ey分量中,缺失率也是以单个分量为单位进行设置。从结果(图17)可知,数据的噪声占比与去噪精度成明显的反比关系,当噪声占比10%时,r最高可达0.99左右;当噪声占比增加到20%时,r仅为0.96左右,下降较为明显;当占比增加到25%时,r曲线随着训练的进行不增反降。这是因为噪声所破坏的信号占比越大,其数据特征也丢失越多,造成模型无法准确学习数据的整体特征,进而导致收敛方向偏差,造成精度不增反降。综上,为保证处理效果,当使用本文方法去噪时,建议每个分量的噪声占比不大于20%。
2)连续干扰长度的影响
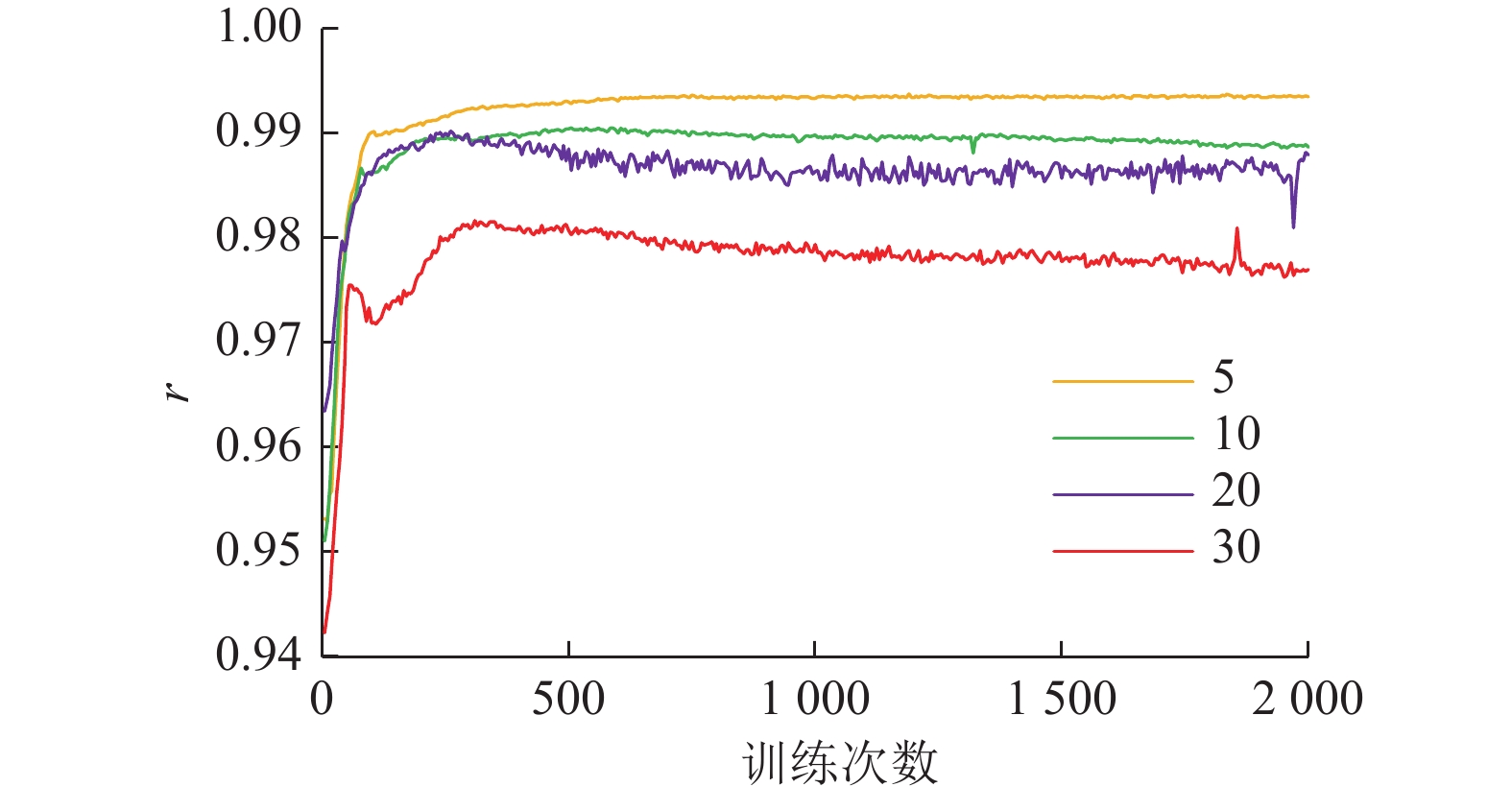
与此同时,在相同的缺失率下,不同的噪声连续干扰长度也会对处理效果产生影响。一般情况下,连续干扰长度越长则恢复的难度也越大。本文对连续干扰长度分别为5、10、20、30个采样点的样本进行了试验(图18),各样本总的噪声占比均为10%,同样假设噪声只存在于Ex及Ey分量中。试验结果表明:去噪精度与连续干扰的长度是成反比的,当连续干扰长度为5个点时,r曲线稳定连续,平稳增加;当长度为10个点时,r有一定减小,r曲线平稳度尚可,但在1 500次训练后,曲线呈现出了明显的下探趋势;当连续干扰长度达到20和30个采样点时,500次训练后r出现了明显的下降,且曲线波动较大,这是由于过长的连续缺失阻碍了模型对于数据整体特征的把握,导致收敛方向振荡所致。综上,建议在使用本文方法处理脉冲类噪声时,噪声的连续干扰长度不大于10个采样点。
总的来看,在4分量大地电磁数据中,当单个分量噪声占比不大于20%,且连续长度不超过10个采样点时,BRITS方法的去噪精度较高。为后续的研究提供了新思路和实验基础。
6 结 论
(1) 将大地电磁数据中脉冲类噪声处理问题转换为时间序列插补问题,并提出了基于BRITS模型的处理方法,为大地电磁数据处理引入新思路。
(2) 相比传统的EMD阈值传统时频域去噪技术,该方法在脉冲类噪声处理精度、有效信号保留能力上更具优势。且BRITS模型具有以数据驱动的特点,使得模型能够自主提取大地电磁不同分量及不同时刻之间的关系特征,有效减少了主观因素影响。
(3) 该方法可通过学习大地电磁不同分量不同时刻的关系特征,对于处理其他与脉冲类噪声特征相似的短时间、突变性噪声也有一定的参考价值。
(4) 该方法依赖待处理数据构建数据集并进行模型训练,尚不支持批量化处理,处理效率及模型的泛化能力有待进一步提升。
符号注释:
${{\boldsymbol{b}}_h}$ 、${{\boldsymbol{b}}_x}$ 、${{\boldsymbol{b}}_{\textit{z}}}$ 、${{\boldsymbol{b}}_\beta }$ 、${{\boldsymbol{b}}_\gamma }$ 均为偏置向量;${\hat {\boldsymbol{c}}_t}$ 为t时刻经过对${\hat {\boldsymbol{x}}_t}$ 与${\hat {{{{\textit{z}}}}}_t}$ 权值分配后的最终估计向量;${\boldsymbol{c}}_t^c$ 为t时刻的最终补全向量;D为特征维度;f(n)为离散时间序列;g(n)为离散时间序列;Ex、Ey分别为电场x、y方向的分量;Hx、Hy分别为磁场x、y方向的分量;${{\boldsymbol{h}}_t}$ 为t时刻的隐藏层;${\boldsymbol{m}}_t^d$ 为t时刻第d个维度的掩码向量值;m为掩码向量;${{\boldsymbol{m}}_t}$ 为t时刻的掩码向量;median表示求取中位数;r为归一化互相关系数;N为时间序列数据长度;${s_t}$ 为t时刻的时间值;RS/N为信噪比,dB;t为某一时刻;T为截取脉冲类噪声的阈值;${{\boldsymbol{U}}_h}$ 、${{\boldsymbol{W}}_h}$ 、${{\boldsymbol{W}}_x}$ 、${{\boldsymbol{W}}_\beta }$ 、${{\boldsymbol{W}}_\gamma }$ 均为权重矩阵;${{\boldsymbol{W}}_{\textit{z}}}$ 为对角元素为0的权重矩阵;x为删除噪声干扰段的大地电磁时间序列数据;xmean为删除噪声干扰段的大地电磁时间序列数据平均值;xmax为删除噪声干扰段的大地电磁时间序列数据最大值;xnorm为删除噪声干扰段的大地电磁时间序列数据归一化值;${\boldsymbol{x}}_t^{}$ 为t时刻的时间序列;${\hat {\boldsymbol{x}}_t}$ 为t时刻的估计值;${\boldsymbol{x}}_t^c$ 为t时刻的补全向量;X为多维时间序列;Xmiss为含缺失值的多维时间序列;ynorm_pred为网络输出的归一化预测值;ypred为大地电磁时间序列数据插补结果;${\hat {{{\boldsymbol{z}}}}_t}$ 为包含特征属性的估计向量;${{\text{β}} _t}$ 为t时刻分配${\hat {\boldsymbol{x}}_t}$ 与${\hat {{{\boldsymbol{z}}}}_t}$ 的权值矩阵,${{\text{β}} _t} \in [0,1]^D $ ;${{\text{γ}} _t}$ 为t时刻的衰减因子;δ为时延向量;${\text{δ}} _t^d$ 为t时刻第d个维度的时延向量值;${ {{\text{δ}}} _t}$ 为t时刻的时延向量;σ为信号的总体稳健性均方误差估计;${\ell _t}$ 为t时刻的网络损失;${\mathcal{L}_e}$ 为平均绝对误差;< >为计算向量内积;$ \odot $ 为矩阵元素对应相乘;○为拼接操作;φ为sigmoid函数。 -
表 1 仿真数据精度对比
Table 1 Comparison of accuracy of different denoising methods for simulated data
类型 r RS/N/dB 仿真数据 0.602 −2.10 EMD阈值方法 0.778 3.09 BRITS方法 0.999 29.97 -
[1] 严家斌. 大地电磁信号处理理论及方法研究[D]. 长沙:中南大学,2003. YAN Jiabin. The study on theory and method of magnetotelluric signal processing[D]. Changsha:Central South University,2003.
[2] 严家斌,刘贵忠. 基于小波变换的脉冲类电磁噪声处理[J]. 煤田地质与勘探,2007,35(5):61−65. DOI: 10.3969/j.issn.1001-1986.2007.05.016 YAN Jiabin,LIU Guizhong. Like-impulse electromagnetic noise processing based wavelet transform[J]. Coal Geology & Exploration,2007,35(5):61−65. DOI: 10.3969/j.issn.1001-1986.2007.05.016
[3] 蔡剑华,汤井田,王先春. 基于经验模态分解的大地电磁资料人文噪声处理[J]. 中南大学学报(自然科学版),2011,42(6):1786−1790. CAI Jianhua,TANG Jingtian,WANG Xianchun. Human noise elimination for magnetotelluric data based on empirical mode decomposition[J]. Journal of Central South University (Science and Technology),2011,42(6):1786−1790.
[4] 王正海,耿欣,姚卓森,等. 基于经验模态分解的MT数据脉冲类电磁噪声处理[J]. 吉林大学学报(地球科学版),2014,44(1):344−349. WANG Zhenghai,GENG Xin,YAO Zhuosen,et al. Like-impulse electromagnetic noise processing based on empirical mode decomposition[J]. Journal of Jilin University (Earth Science Edition),2014,44(1):344−349.
[5] 汤井田,刘子杰,刘峰屹,等. 音频大地电磁法强干扰压制试验研究[J]. 地球物理学报,2015,58(12):4636−4647. DOI: 10.6038/cjg20151225 TANG Jingtian,LIU Zijie,LIU Fengyi,et al. The denoising of the audio magnetotelluric data set with strong interferences[J]. Chinese Journal of Geophysics,2015,58(12):4636−4647. DOI: 10.6038/cjg20151225
[6] 汤井田,李晋,肖晓,等. 数学形态滤波与大地电磁噪声压制[J]. 地球物理学报,2012,55(5):1784−1793. DOI: 10.6038/j.issn.0001-5733.2012.05.036 TANG Jingtian,LI Jin,XIAO Xiao,et al. Mathematical morphology filtering and noise suppression of magnetotelluric sounding data[J]. Chinese Journal of Geophysics,2012,55(5):1784−1793. DOI: 10.6038/j.issn.0001-5733.2012.05.036
[7] 汤井田,李晋,肖晓,等. 基于数学形态滤波的大地电磁强干扰分离方法[J]. 中南大学学报(自然科学版),2012,43(6):2215−2221. TANG Jingtian,LI Jin,XIAO Xiao,et al. Magnetotelluric sounding data strong interference separation method based on mathematical morphology filtering[J]. Journal of Central South University (Science and Technology),2012,43(6):2215−2221.
[8] 汤磊,汤洪志,韩雪平,等. 数学形态滤波在音频大地电磁去噪中的应用[J]. 工程地球物理学报,2013,10(4):533−538. DOI: 10.3969/j.issn.1672-7940.2013.04.018 TANG Lei,TANG Hongzhi,HAN Xueping,et al. The application of mathematical morphological filtering to the noise suppression of audio magnetotelluric sounding data[J]. Chinese Journal of Engineering Geophysics,2013,10(4):533−538. DOI: 10.3969/j.issn.1672-7940.2013.04.018
[9] 李晋,张贤,蔡锦. 利用变分模态分解(VMD)和匹配追踪(MP)联合压制音频大地电磁(AMT)强干扰[J]. 地球物理学报,2019,62(10):3866−3884. DOI: 10.6038/cjg2019M0407 LI Jin,ZHANG Xian,CAI Jin. Suppression of strong interference for AMT using VMD and MP[J]. Chinese Journal of Geophysics,2019,62(10):3866−3884. DOI: 10.6038/cjg2019M0407
[10] 李晋,燕欢,汤井田,等. 基于匹配追踪和遗传算法的大地电磁噪声压制[J]. 地球物理学报,2018,61(7):3086−3101. DOI: 10.6038/cjg2018L0229 LI Jin,YAN Huan,TANG Jingtian,et al. Magnetotelluric noise suppression based on matching pursuit and genetic algorithm[J]. Chinese Journal of Geophysics,2018,61(7):3086−3101. DOI: 10.6038/cjg2018L0229
[11] 舒幸宁. 基于AR模型的大地电磁脉冲噪声压制[J]. 科技创新与应用,2021,11(18):102−104. SHU Xingning. Suppression of magnetotelluric pulse noise based on AR model[J]. Technology Innovation and Application,2021,11(18):102−104.
[12] 汤井田,李广,周聪,等. 基于字典学习的音频大地电磁数据处理[J]. 地球物理学报,2018,61(9):3835−3850. DOI: 10.6038/cjg2018L0376 TANG Jingtian,LI Guang,ZHOU Cong,et al. Denoising AMT data based on dictionary learning[J]. Chinese Journal of Geophysics,2018,61(9):3835−3850. DOI: 10.6038/cjg2018L0376
[13] 桂团福,邓居智,李广,等. 数学形态学和K-SVD字典学习在大地电磁数据去噪中的应用[J]. 中国有色金属学报,2021,31(12):3713−3729. GUI Tuanfu,DENG Juzhi,LI Guang,et al. De-noising magnetotelluric data based on mathematical morphology and K-SVD dictionary learning[J]. The Chinese Journal of Nonferrous Metals,2021,31(12):3713−3729.
[14] 王昊,严加永,付光明,等. 深度学习在地球物理中的应用现状与前景[J]. 地球物理学进展,2020,35(2):642−655. DOI: 10.6038/pg2020CC0476 WANG Hao,YAN Jiayong,FU Guangming,et al. Current status and application prospect of deep learning in geophysics[J]. Progress in Geophysics,2020,35(2):642−655. DOI: 10.6038/pg2020CC0476
[15] 韩盈,安志国,屈文璋. 基于机器学习的大地电磁时域数据处理的研究现状[J]. 地球物理学进展,2021,36(5):1975−1987. DOI: 10.6038/pg2021EE0581 HAN Ying,AN Zhiguo,QU Wenzhang. Research status of magnetotelluric time domain data processing based on machine learning[J]. Progress in Geophysics,2021,36(5):1975−1987. DOI: 10.6038/pg2021EE0581
[16] YU Siwei,MA Jianwei. Deep learning for geophysics:Current and future trends[J]. Reviews of Geophysics,2021,59(3):e2021RG000742. DOI: 10.1029/2021RG000742
[17] 许滔滔,王中兴,肖卓伟,等. 基于LSTM循环神经网络的大地电磁工频干扰压制[J]. 地球物理学进展,2020,35(5):2016−2022. DOI: 10.6038/pg2020DD0402 XU Taotao,WANG Zhongxing,XIAO Zhuowei,et al. Magnetotelluric power frequency interference suppression based on LSTM recurrent neural network[J]. Progress in Geophysics,2020,35(5):2016−2022. DOI: 10.6038/pg2020DD0402
[18] 王斯昊,何兰芳. 基于LSTM循环网络的MT时间序列去噪可行性分析[C]//中国地球物理学会,地球物理技术委员会. 第九届学术会议—全域地球物理探测与智能感知学术研讨会会议摘要集. 武汉,2021:355–357. [19] 汪凯翔,黄清华,吴思弘. 长短时记忆神经网络在地电场数据处理中的应用[J]. 地球物理学报,2020,63(8):3015−3024. DOI: 10.6038/cjg2020O0119 WANG Kaixiang,HUANG Qinghua,WU Sihong. Application of long short-term memory neural network in geoelectric field data processing[J]. Chinese Journal of Geophysics,2020,63(8):3015−3024. DOI: 10.6038/cjg2020O0119
[20] 杨凯,唐卫东,刘诚,等. 基于LSTM循环神经网络的大地电磁方波噪声抑制[J]. 物探与化探,2022,46(4):925−933. YANG Kai,TANG Weidong,LIU Cheng,et al. Suppression of magnetotelluric square wave noise based on a LSTM recurrent neural network[J]. Geophysical and Geochemical Exploration,2022,46(4):925−933.
[21] CAO Wei,WANG Dong,LI Jian,et al. BRITS:bidirectional recurrent imputation for time series[C]//Proceedings of the 32nd International Conference on Neural Information Processing Systems. Cambridge:MIT Press,2018:6776–6786.
[22] XIE Chuanjie,HUANG Chong,ZHANG Deqiang,et al. BiLSTM-I:A deep learning-based long interval gap-filling method for meteorological observation data[J]. International Journal of Environmental Research and Public Health,2021,18(19):10321. DOI: 10.3390/ijerph181910321
[23] 郑欣彤,边婷婷,张德强,等. ARIMA和LSTM方法长时间温度观测数据缺失值插补的比较[J]. 计算机应用,2022,42(增刊1):130−135. ZHENG Xintong,BIAN Tingting,ZHANG Deqiang,et al. Comparison of ARIMA and LSTM methods for interpolation of missing values of long-time temperature observations[J]. Journal of Computer Applications,2022,42(Sup.1):130−135.
[24] 朱政. 雷达高分辨距离像缺失信号重构与目标识别方法研究[D]. 西安:西安电子科技大学,2022. ZHU Zheng. Research on reconstruction and target recognition of missing signal of radar high resolution range profile[D]. Xi’an:Xidian University,2022.
[25] 严家斌,刘贵忠. 天然大地电磁场时间序列的分形特征[J]. 煤田地质与勘探,2007,35(2):66−69. DOI: 10.3969/j.issn.1001-1986.2007.02.020 YAN Jiabin,LIU Guizhong. Fractal characteristic of time series of nature field[J]. Coal Geology & Exploration,2007,35(2):66−69. DOI: 10.3969/j.issn.1001-1986.2007.02.020
[26] 王辉,魏文博,金胜,等. 基于同步大地电磁时间序列依赖关系的噪声处理[J]. 地球物理学报,2014,57(2):531−545. DOI: 10.6038/cjg20140218 WANG Hui,WEI Wenbo,JIN Sheng,et al. Removal of magnetotelluric noise based on synchronous time series relationship[J]. Chinese Journal of Geophysics,2014,57(2):531−545. DOI: 10.6038/cjg20140218
[27] WANG Hui,CAMPANYÀ J,CHENG Jiulong,et al. Synthesis of natural electric and magnetic Time-series using Inter-station transfer functions and time-series from a Neighboring site (STIN):Applications for processing MT data[J]. Journal of Geophysical Research:Solid Earth,2017,122(8):5835−5851. DOI: 10.1002/2017JB014190
[28] 王书明,王家映. 大地电磁信号统计特征分析[J]. 地震学报,2004,26(6):669−674. DOI: 10.3321/j.issn:0253-3782.2004.06.013 WANG Shuming,WANG Jiaying. Analysis on statistic characteristics of magnetotelluric signal[J]. Acta Seismologica Sinica,2004,26(6):669−674. DOI: 10.3321/j.issn:0253-3782.2004.06.013
[29] NEUKIRCH M,GARCIA X. Nonstationary magnetotelluric data processing with instantaneous parameter[J]. Journal of Geophysical Research:Solid Earth,2014,119(3):1634−1654. DOI: 10.1002/2013JB010494
[30] UHM J,HEO J,MIN D J,et al. Imaging strategies to interpret 3-D noisy audio-magnetotelluric data acquired in Gyeongju,South Korea:Data processing and inversion[J]. Geophysical Journal International,2021,225(2):744−758. DOI: 10.1093/gji/ggab002
[31] YANG Yue,WANG Xueqiu,HAN Jiangtao,et al. Magnetotelluric transfer function distortion assessment using Nyquist diagrams[J]. Journal of Applied Geophysics,2019,160:218−228. DOI: 10.1016/j.jappgeo.2018.11.018
-
期刊类型引用(1)
1. 翁蒙婷. 基于机器视觉的噪声处理技术研究. 电声技术. 2024(11): 10-12 .  百度学术
百度学术
其他类型引用(0)







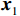
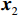
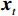
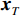
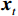

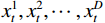
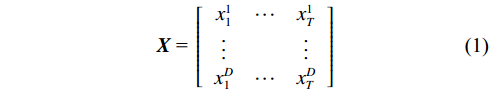
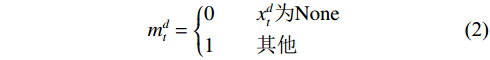
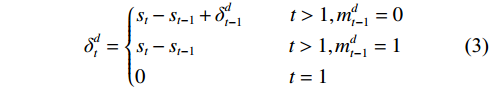
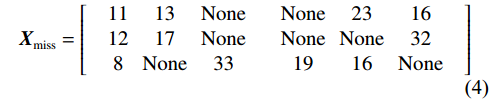
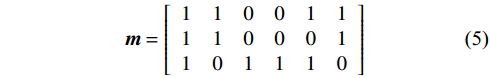
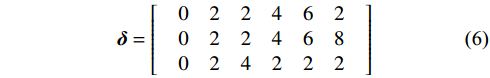


 下载:
下载: